Discover the Magic of Elasticity and Hooke’s Law

Last Updated on November 15, 2022 by Sara Assem

A true wonder of humanity’s creative mind and engineering is the spring! With its many different forms and specific functions—the coil spring, the compression spring, the extension spring, the torsion spring, and more—our mankind was offered the chance to fabricate and invent many more useful objects.

This man-made revolution of tools and applications came out as a consequence of the Scientific Revolution that took place during the 17th and the 18th centuries. Springs undergo a definite physics law, scientifically known as: Hooke’s law of stress-strain, Hooke’s law of elasticity, or simply Hooke’s law.

What is Hooke’s law? Does it apply to elastic materials or to inelastic materials? How does Hooke’s law work? And why is Hooke’s law important in physics and in our lives in general? Those are all questions that might all have come to your mind the moment you read the word “Hooke’s law.”

In this fruitful blog, Hooke’s law will be explained extensively and you will grasp its nature, know what its examples and applications are, understand which mathematical equations describe it, and grasp how to account for it… Let’s go!

 

Try Hooke’s Law Experiment Now!

 

What’s the Story of Hooke’s Law Spring?

The spring’s ability to successfully store mechanical energy in addition to its elasticity—the property that causes the material/object to be restored to its original shape after distortion—allowed it to be widely used in a variety of applications. These applications vary from the famous Slinky to pendulum clocks, wind-up toys, rat traps, digitized micromirror devices, automotive suspension systems, hand shears, and watches.

Before any newly invented device is competent to be used in many applications, we’re required to truly understand the mechanics behind it, at least the very basics. And here, speaking of springs, elasticity, force, and torsion are the properties we need to address. Together, they form what is called Hooke’s Law.

In 1676, the English physicist Robert Hooke stated the law, by demonstrating a relation between the spring’s elasticity and the forces applied on it. While the law is related to elasticity and the forces, it is also related to the distance of the extension or the compression. A musician plucking the strings of his guitar or the amount of wind blowing against a tall building to make it bend and sway are considered examples of Hooke’s law where those elastic bodies are, to some extent, deformed.

Generalized Hooke’s Law

Before delving into the details and calculations of Hooke’s law, you must know that it is only a first-order linear approximation of springs’ real response–as well as other elastic bodies–to the forces applied on them. Materials cannot be stretched beyond a maximum size, and they also cannot be compressed exceeding a certain limit.

In other words, this linear law fails whenever the applied forces exceed the allowed deformation limits. These limits are known as the elastic limits, whereas most materials will face a change in state, or a permanent deformation when they reach those limits. For most solid bodies and small deformations, Hooke’s law is a precise approximation that describes the process quite well. That is, the law is actively and widely used in almost all engineering and science branches.

This law stands as the foundational principle for many applications; such as: the mechanical clock’s balance wheel, the galvanometer, the manometer (aka. the pressure gauge), and the spring scale, while it also governs many disciplines around us, such as acoustics, molecular mechanics, and seismology.

A generalization of Hooke’s law was successfully achieved by the modern theory of elasticity. The theory identifies both the stress applied on an elastic material/object and the strain (the deformation) to be proportional to each other.

However, the proportionality factor might not be expressed as a single real number, but rather as a tensor, since general stresses and strains can have multiple independent components. A tensor is a form of mathematical expressions, which is represented by a matrix of real numbers.

Using this general form of Hooke’s law, it is easy to deduce the stress-strain relation for complex shapes of objects. This is based on studying the intrinsic properties of the object’s materials. A rod that is homogeneous and with a uniform cross-section will behave in the same way a simple spring will whenever it is stretched. Such a relation connects the stiffness (k) that is directly proportional to the rod’s cross-sectional area, and inversely proportional to its length.

 

For FREE ! Create Account To Try Hook's Law Experiment

Hooke’s Law Experiment

Since laws and theories need experiments to verify whether they are right or wrong, Hooke’s law was proven by experiment to be magically fit and verified. Hooke’s law experiment is a simple one to perform, with its handy setup and mindful concept. It is the ease of carrying this experiment that makes it chosen to be conducted in any foundation year’s physics lab. Now, can you guess its concept of work and delivery scope?

Hooke’s law experiment focuses on treating elastic bodies, hence studying their elasticity and how they adhere and respond to deformations. The experiment’s setup consists of a coil spring and interchangeable weights that are suspended from it. The spring’s change in length is proportional to the force of gravity, denoted as F, that affects the suspended weight.

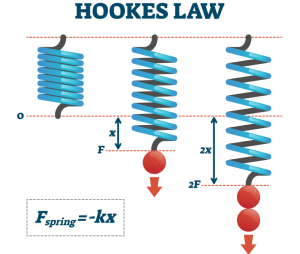
The experiment can rather be conducted using one coil spring and many weights, or using different coil springs and different weights. In all the cases, we study the coil’s elongation – the change in length – in response to the weights in use. Wisely choosing the diameter of both the wire and the coil will result in a noticeable verification of Hooke’s law.

Learn Hooke’s Law Equation

In order to analytically grasp how Hooke’s law experiment graphs and data will look like, we firstly need to acquire a certain knowledge of the equation governing Hooke’s law itself.

The extension in the length of the coil spring is what we measure in this experiment, in order to deduce the value of what is scientifically known as the spring’s constant, denoted as k.

Hooke’s law formula is given as follows:

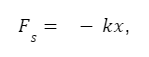
where

Fs: the applied gravitational force caused by the weights (measured in Newtons, or m*g),
k: proportionality constant of elasticity that depends on the spring’s material and dimensions (measured in Newtons/meter), and
x: compression or stretch of the spring from its equilibrium position (measured in meters).

One more important note to consider while recording your measurements is to keep an eye on Hooke’s law units mentioned before. Hooke’s law equation is vital in many applications and examples that we interact with on a regular basis and throughout our everyday life.

The law applies when a straight beam of concrete (similar to that used in buildings) or a steel bar, supported at both ends, is bent by a weight that is placed at an intermediate point along it. In this case, the beam’s deviation is equal to the displacement (x), and this is considered as a Hooke’s law example.

Hooke’s Law Graph

Logically, the graph of Hooke’s law experiment must be related to the previously mentioned terms and characteristics. Hence, we record readings of the following:

1. The mass (in kilograms)
2. Length of the spring (in meters)
3. Elongation (in meters)

An example of the data table is shown in the figure below.

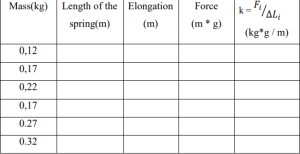
Hooke’s law calculator includes two more mathematical calculations that are based on those readings. The calculations are:

1. The force, which equals the mass times the gravitational acceleration (given in Newtons, or kg*m/s²)
2. The spring’s constant (in Newtons/meter)

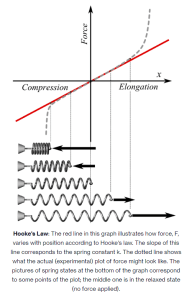
Our desired value of the spring’s constant is determined by drawing a graph of the force on the y-axis, and the spring’s displacement from the equilibrium on the x-axis. This results in a straight line, and it only falls off when exceeding the plastic limit. Calculating the graph’s slope, we easily get the spring’s constant. The larger the spring constant, k, the more force the spring applies per amount of displacement.

The graph, for either compression or extension, is drawn as follows:

Now, you can easily conduct Hooke’s Law on your own for free, and learn more about the law’s applications and rules only on PraxiLabs Virtual Labs!